Preface ....................................................... vii
1 Banach algebras and uniform algebras ......................... 1
1.1 Commutative Banach algebras ............................. 1
1.2 Uniform algebras ........................................ 7
1.3 Inductive and inverse limits of algebras and sets ...... 18
1.4 Bourgain algebras of commutative Banach algebras ....... 24
1.5 Polynomial extensions of Banach algebras ............... 32
1.6 Isomorphisms between uniform algebras .................. 39
1.7 Notes .................................................. 54
2 Three classical families of functions ....................... 57
2.1 Almost periodic functions of one and several
variables .............................................. 57
2.2 Harmonic functions in the unit disc .................... 60
2.3 The Poisson integral in the unit disc .................. 66
2.4 Classes of harmonic functions in the unit disc ......... 71
2.5 Notes .................................................. 74
3 Groups and semigroups ....................................... 77
3.1 Topological groups and their duals ..................... 77
3.2 Functions and measures on groups ....................... 84
3.3 Bochner-Fejér operators on groups ...................... 91
3.4 Semigroups and semicharacters .......................... 95
3.5 The set of semicharacters ............................. 101
3.6 The semigroup algebra 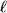 1 (S) of a semigroup ........... 109
3.7 Notes ................................................. 115
4 Shift-invariant algebras on compact groups ................. 117
4.1 Algebras of S-functions on groups ..................... 117
4.2 The maximal ideal space of a shift invariant
algebra ............................................... 121
4.3 Automorphisms of shift-invariant algebras ............. 130
4.4 p-groups and peak groups of shift-invariant
algebras .............................................. 132
4.5 Radó's and Riemann's theorems on G-discs .............. 140
4.6 Asymptotically almost periodic functions in one
variable .............................................. 144
4.7 Notes ................................................. 148
5 Extension of semicharacters and additive weights ........... 151
5.1 Extension of non-vanishing semicharacters ............. 151
5.2 Extension of additive weights and semicharacters on
semigroups ............................................ 155
5.3 Semigroups with extendable additive weights ........... 159
5.4 Weights on algebras generated by Archimedean ordered
semigroups ............................................ 170
5.5 Notes ................................................. 176
6 G-disc algebras ............................................ 177
6.1 Analytic functions on groups and G-discs .............. 177
6.2 Bourgain algebras of G-disc algebras .................. 181
6.3 Orthogonal measures to G-disc algebras ................ 184
6.4 Primary ideals of G-disc algebras ..................... 191
6.5 Notes ................................................. 201
7 Harmonicity on groups and G-discs .......................... 203
7.1 Harmonic functions on groups and G-discs .............. 203
7.2 Lp-harmonicity on groups and G-discs .................. 210
7.3 L1 -harmonic functions on groups and G-discs .......... 218
7.4 The space 1 (S) of a semigroup ........... 109
3.7 Notes ................................................. 115
4 Shift-invariant algebras on compact groups ................. 117
4.1 Algebras of S-functions on groups ..................... 117
4.2 The maximal ideal space of a shift invariant
algebra ............................................... 121
4.3 Automorphisms of shift-invariant algebras ............. 130
4.4 p-groups and peak groups of shift-invariant
algebras .............................................. 132
4.5 Radó's and Riemann's theorems on G-discs .............. 140
4.6 Asymptotically almost periodic functions in one
variable .............................................. 144
4.7 Notes ................................................. 148
5 Extension of semicharacters and additive weights ........... 151
5.1 Extension of non-vanishing semicharacters ............. 151
5.2 Extension of additive weights and semicharacters on
semigroups ............................................ 155
5.3 Semigroups with extendable additive weights ........... 159
5.4 Weights on algebras generated by Archimedean ordered
semigroups ............................................ 170
5.5 Notes ................................................. 176
6 G-disc algebras ............................................ 177
6.1 Analytic functions on groups and G-discs .............. 177
6.2 Bourgain algebras of G-disc algebras .................. 181
6.3 Orthogonal measures to G-disc algebras ................ 184
6.4 Primary ideals of G-disc algebras ..................... 191
6.5 Notes ................................................. 201
7 Harmonicity on groups and G-discs .......................... 203
7.1 Harmonic functions on groups and G-discs .............. 203
7.2 Lp-harmonicity on groups and G-discs .................. 210
7.3 L1 -harmonic functions on groups and G-discs .......... 218
7.4 The space 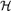 P( P(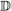 G) as Banach algebra .................... 221
7.5 Fatou type theorems for families of harmonic
measures on groups .................................... 223
7.6 Notes ................................................. 227
8 Shift-invariant algebras and inductive limit algebras on
groups ..................................................... 229
8.1 Inductive limits of H∞-algebras ....................... 229
8.2 Blaschke inductive limits of disc algebras ............ 236
8.3 Blaschke inductive limit algebras of annulus type ..... 240
8.4 Parts of Blaschke inductive limit algebras ............ 247
8.5 H∞-type spaces on compact groups ...................... 254
8.6 Bourgain algebras of inductive limit algebras on
groups ................................................ 257
8.7 Notes ................................................. 261
Bibliography .................................................. 263
Index ......................................................... 271 G) as Banach algebra .................... 221
7.5 Fatou type theorems for families of harmonic
measures on groups .................................... 223
7.6 Notes ................................................. 227
8 Shift-invariant algebras and inductive limit algebras on
groups ..................................................... 229
8.1 Inductive limits of H∞-algebras ....................... 229
8.2 Blaschke inductive limits of disc algebras ............ 236
8.3 Blaschke inductive limit algebras of annulus type ..... 240
8.4 Parts of Blaschke inductive limit algebras ............ 247
8.5 H∞-type spaces on compact groups ...................... 254
8.6 Bourgain algebras of inductive limit algebras on
groups ................................................ 257
8.7 Notes ................................................. 261
Bibliography .................................................. 263
Index ......................................................... 271
|