Annales academiae scientiarum Fennicae. Mathematica dissertationes. Vol.153: Boundedness of weakly singular integral operators on domains / Vähäkangas A.V. - Helsinki: Suomalainen tiedeakatemia, 2009. – 111 p. – Ref.: p.109-111. - ISBN 951-41-1034-4; ISSN 1239-6303  Место хранения:   013 | Институт математики СО РАН | Новосибирск | Библиотека

Оглавление / Contents

1. Introduction ................................................. 5 2. WSIO's of convolution type .................................. 13 2.1. Homogeneous Besov spaces ............................... 13 2.2. Boundedness of convolution operators ................... 17 2.3. Unbounded operators .................................... 23 3. WSIO's with global kernels .................................. 27 3.1. Reduced T1 theorem in 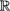n ............................... 27 3.2. BMO-type spaces on domains ............................. 40 3.3. Endpoint estimates for restricted operators ............ 45 3.4. TΧΩ, theorem for restricted operators .................. 51 4. Regularity of standard kernels .............................. 62 4.1. Kernel spaces .......................................... 62 4.2. Dyadic resolution of unity ............................. 64 4.3. Regularity of kernels in uniform domains ............... 71 5. Extension of smooth kernels ................................. 79 5.1. Hölder spaces .......................................... 79 5.2. Uniformity and products ................................ 86 5.3. Atomic decomposition and kernel extension .............. 89 6. WSIO's on domains ........................................... 96 6.1. Admissible domains ..................................... 96 6.2. TΧΩ, theorem revisited ................................. 98 6.3. T1 theorem on admissible domains ....................... 99 Appendix A. Notation .......................................... 102 Appendix B. Compactly supported wavelets ...................... 105 Appendix C. Whitney decomposition ............................. 107 References .................................................... 109